Fantastic Info About What Does A Smooth Graph Look Like Line Organizational Structure Example

It reduces the noise to emphasize the signal that can contain trends and cycles.

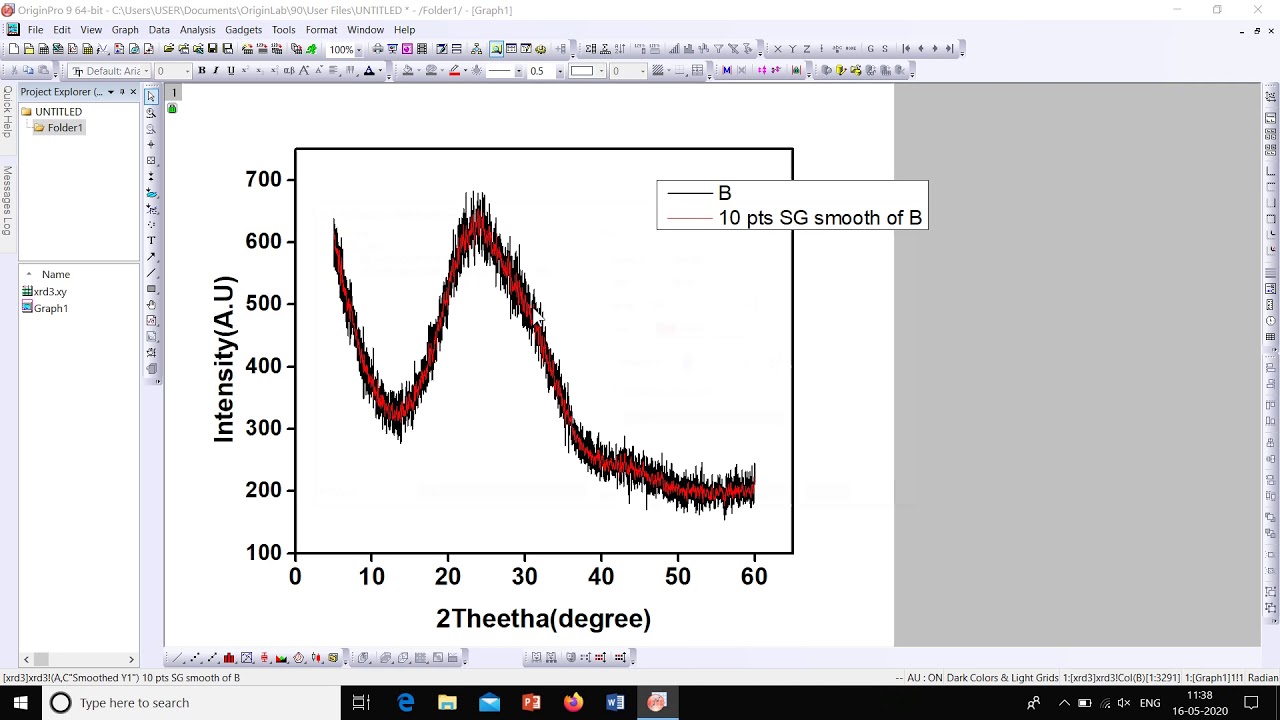
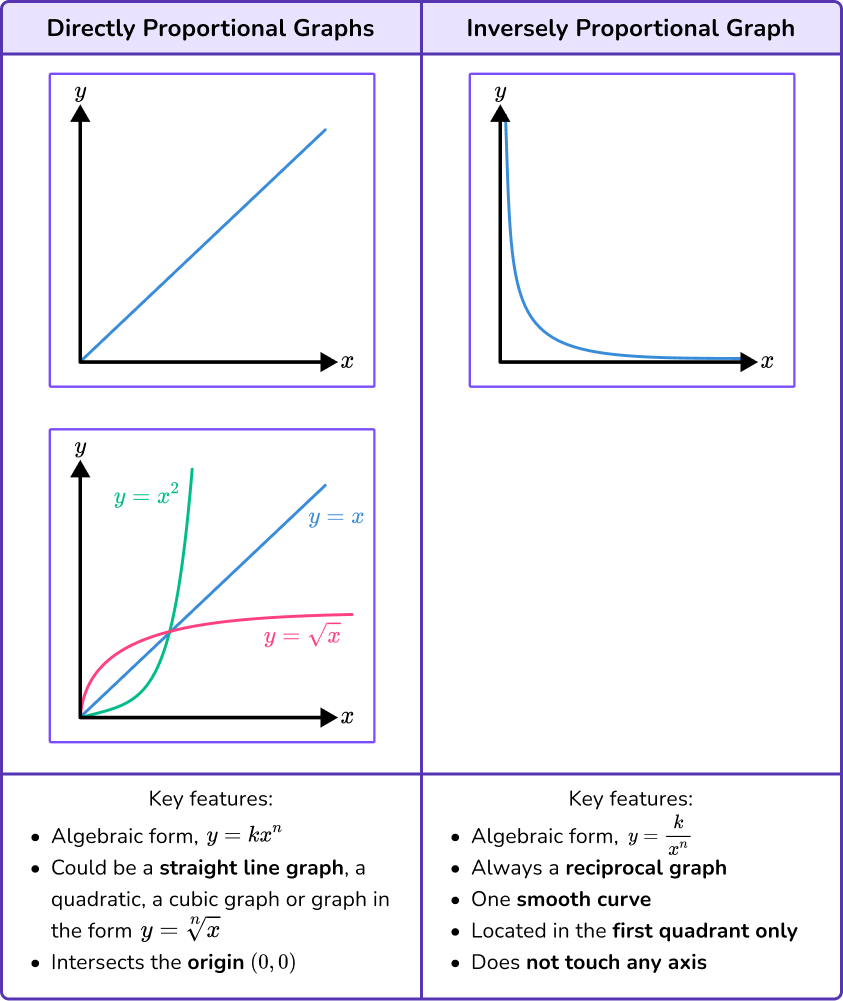
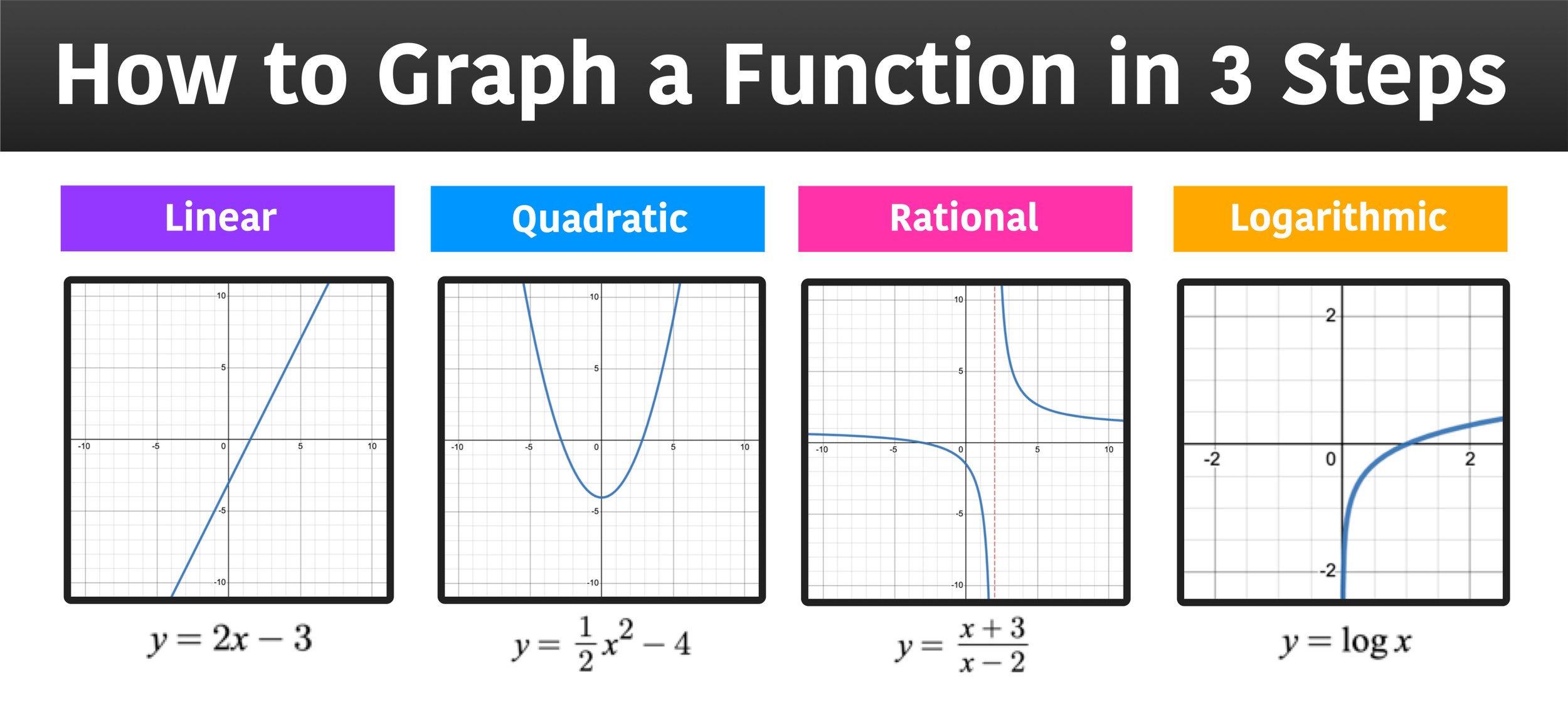
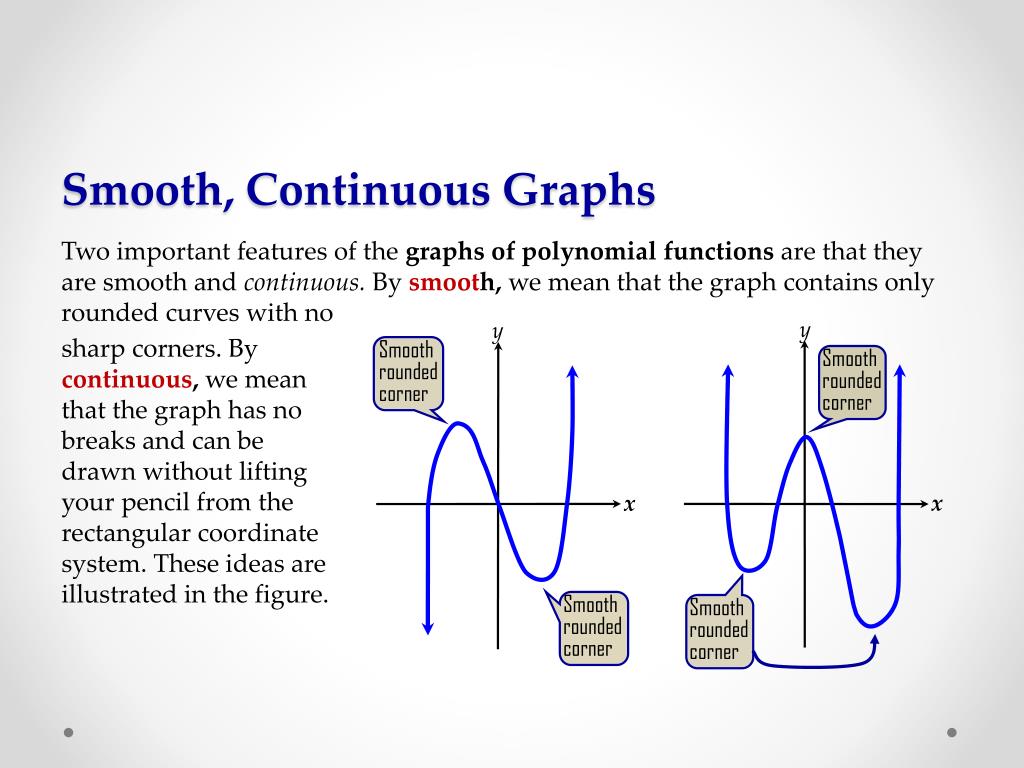
What does a smooth graph look like. It is a curve in the real plane parametrized $f:t\to (t^2,t^3)$. Simplify your dataset to 10 examples that you know your. Of course, the mapping $f$ is smooth (of any order), and the graph of $f$ is a smooth manifold in $\mathbb{r}^3$, but its image is singular:

Make sure your group of data is displayed in a clean and tidy manner. Select the entire data cell, choose insert, and select chart. Graph functions, plot points, visualize algebraic equations, add sliders, animate graphs, and more.

These computational graphs are made up of vertices (think neurons) for the compute elements, connected by edges (think synapses), which describe the communication paths between vertices. A key assumption of correlation, linear regression and nonlinear regression is that the data are independent of each other. If training looks unstable, as in this plot, then reduce your learning rate to prevent the model from bouncing around in parameter space.

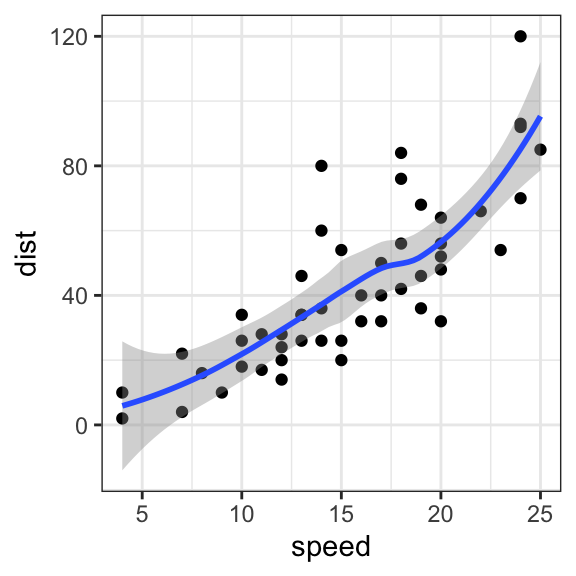
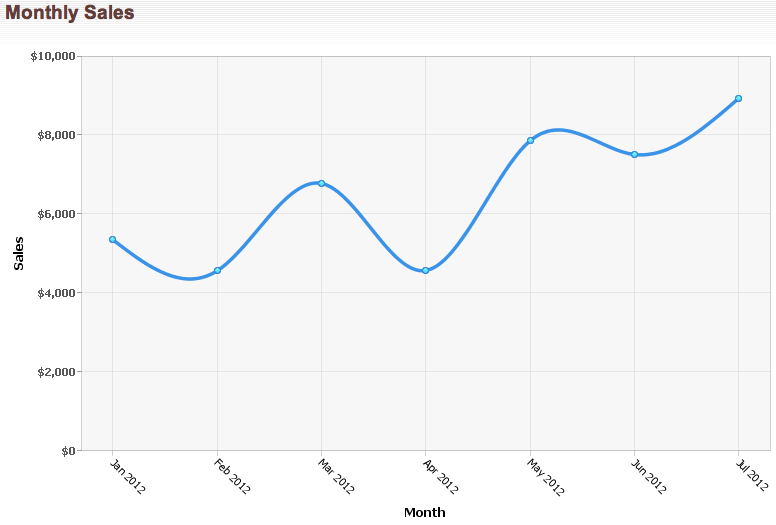
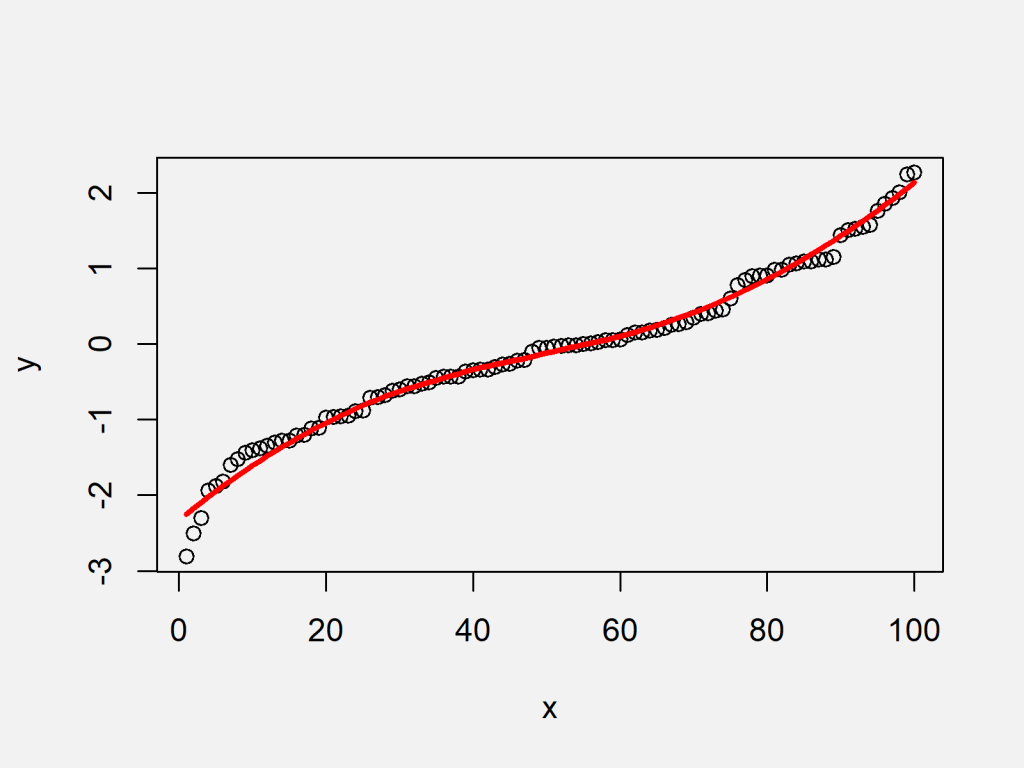
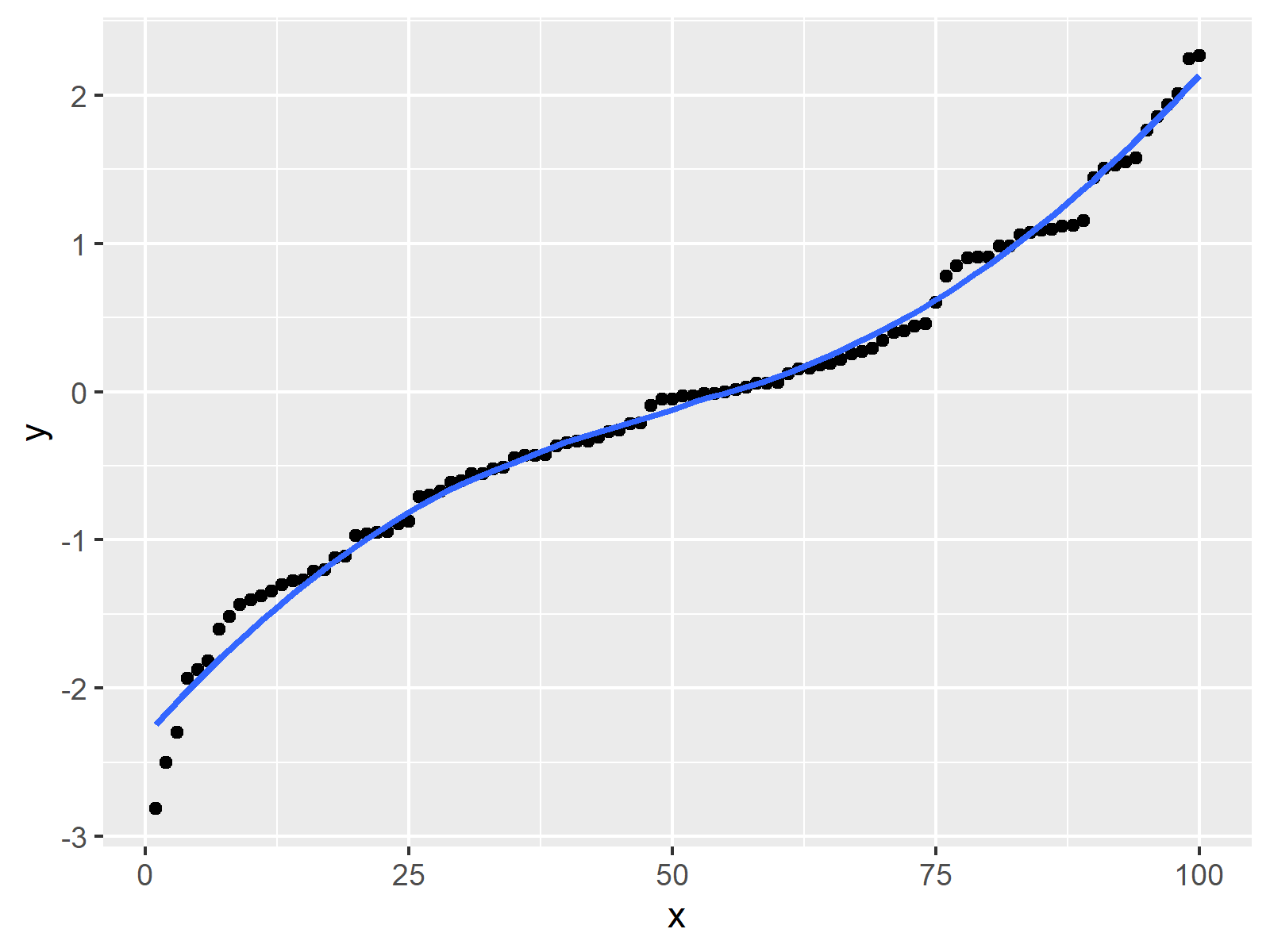
For example, the following graph is smooth: It also appears to be the the approach that ggplot takes. Graph functions, plot points, visualize algebraic equations, add sliders, animate graphs, and more.

Explore math with our beautiful, free online graphing calculator. However, all sources tell that a function is smooth if it has derivatives of all orders. I don't think this will help you too much.

Where smooth atlas means that the transition functions defiened on overlapping charts are smooth from rn to rn. We link the derivative to the local shape of. Graph functions, plot points, visualize algebraic equations, add sliders, animate graphs, and more.

As far as i've understand a smooth manifold is a pair of a manifold and a smooth atlas. It is the zero set $x^3=y^2$. A curve ,let's say $(x(t),y(t))$ is said to be smooth if $x'(t)$ and $y'(t)$ both exist and are continuous.(am i not right?) a function differentiable at a point intuitively means that its graph on coordinate plane has a unique tangent(no corner) at that point.

Definition (smooth curve) let $\gamma\colon [a,b] \to \mathbb{r}^2$ a curve (continous function). It is a curve in the real plane parametrized $f:t\to (t^2,t^3)$. In particular a chart in a smooth atlas is (in some sense) only a homeomorphism.

This one is not smooth: Look at the standard example: By default, google sheet will use the selected group of data to generate a line chart.

This will help us to create a smooth line chart easily. Much better results can be achieved with d3.js. When we draw a graph, networkx uses matplotlib “under the hood.” this is fine for a small graph like this, but if the graph contains 1000+ nodes, matplotlib becomes painfully slow.